Aykut Argun – Page 3 – Soft Matter Lab

Digital Video Microscopy Enhanced by Deep Learning published in Optica

Digital video microscopy enhanced by deep learning
(Cover article)
Saga Helgadottir, Aykut Argun & Giovanni Volpe
Optica 6(4), 506—513 (2019)
doi: 10.1364/OPTICA.6.000506
arXiv: 1812.02653
GitHub: DeepTrack

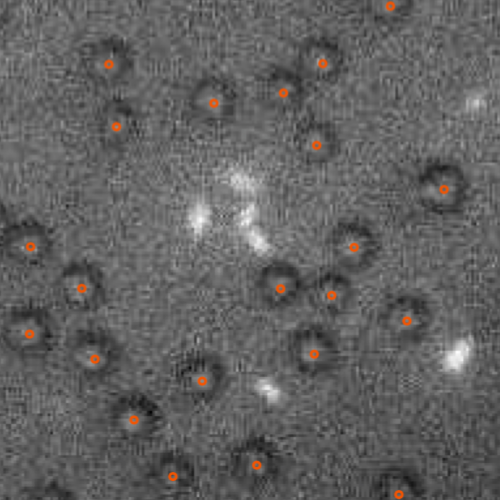
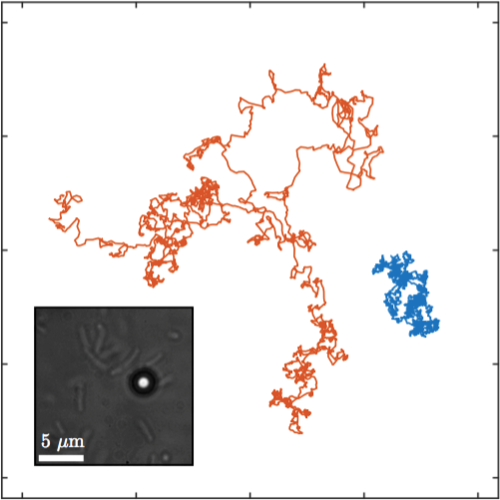
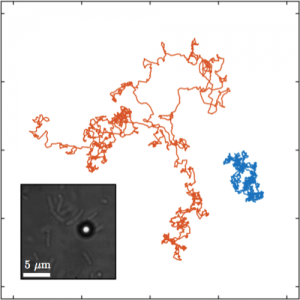
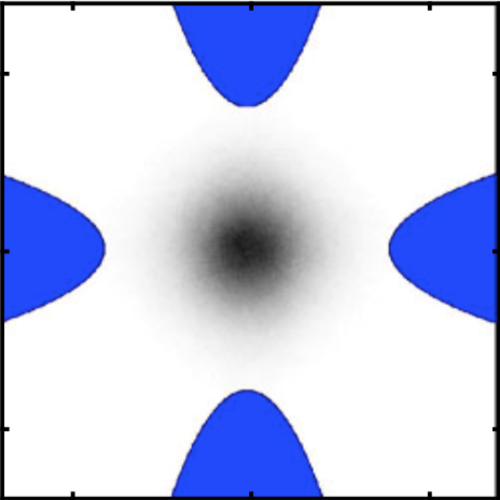
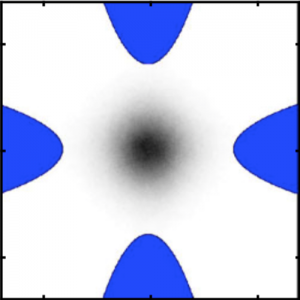
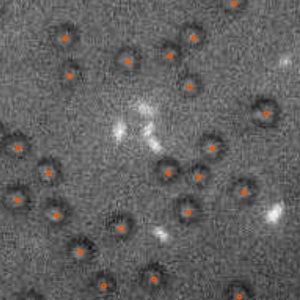
Single particle tracking is essential in many branches of science and technology, from the measurement of biomolecular forces to the study of colloidal crystals. Standard methods rely on algorithmic approaches; by fine-tuning several user-defined parameters, these methods can be highly successful at tracking a well-defined kind of particle under low-noise conditions with constant and homogenous illumination. Here, we introduce an alternative data-driven approach based on a convolutional neural network, which we name DeepTrack. We show that DeepTrack outperforms algorithmic approaches, especially in the presence of noise and under poor illumination conditions. We use DeepTrack to track an optically trapped particle under very noisy and unsteady illumination conditions, where standard algorithmic approaches fail. We then demonstrate how DeepTrack can also be used to track multiple particles and non-spherical objects such as bacteria, also at very low signal-to-noise ratios. In order to make DeepTrack readily available for other users, we provide a Python software package, which can be easily personalized and optimized for specific applications.

Featured in :
“Deep Learning for Particle Tracking”, Optics & Photonics News (December 1, 2019)

Funding:

H2020 European Research Council (ERC) Starting Grant ComplexSwimmers (677511).

Video 1:

Video 2:

Video 3:

Video 4:

Video 5:

Video 6:

Video 7:

Video 8:

Talk on optical tweezers by Aykut Argun at Gothenburg PhD Pub. 17 Oct 2018

Aykut Argun will present a popular science talk on the principles and applications of optical tweezers at a PhD-student event called Gothenburg Ph.D. Pub.

Title: Optical tweezers and applications

Abstract: Can objects be moved contact-free only by the power of light?
The answer which deserved a Nobel Prize in Physics last week is yes.
Aykut Argun from GU Physics will present how in the next Ph.D. Pub.

Place: Haket – Bar å sånt, Första långgatan 32, 413 27 Gothenburg
Time: Wednesday, October 17, 2018 at 7 PM – 10 PM

Talk by A. Argun at IONS Scandinavia 2018, Copenhagen, 5-9 Jun 2018

Experimental realization of a minimal microscopic heat engine
Aykut Argun, Jalpa Soni, Lennart Dabelow, Stefano Bo, Giuseppe Pesce,
Ralf Eichborn & Giovanni Volpe
IONS Scandinavia 2018, Copenhagen, Denmark
5-9 June 2018

Abstract: Microscopic heat engines are microscale systems that convert energy flows between heat reservoirs into work or systematic motion. We have experimentally realized a minimal microscopic heat engine. It consists of a colloidal Brownian particle optically trapped in an elliptical potential well and simultaneously coupled to two heat baths at different temperatures acting along perpendicular directions. For a generic arrangement of the principal directions of the baths and the potential, the symmetry of the system is broken, such that the heat flow drives a systematic gyrating motion of the particle around the potential minimum. Using the experimentally measured trajectories, we quantify the gyrating motion of the particle, the resulting torque that it exerts on the potential, and the associated heat flow between the heat baths. We find excellent agreement between the experimental results and the theoretical predictions.

Reference: Argun et al. Experimental realization of a minimal microscopic heat engine. Physical Review E 96(5), 052106 (2017)

Minimal Microscopic Heat Engine published in Phys. Rev. E

Experimental realization of a minimal microscopic heat engine
Aykut Argun, Jalpa Soni, Lennart Dabelow, Stefano Bo, Giuseppe Pesce, Ralf Eichhorn & Giovanni Volpe
Physical Review E 96(5), 052106 (2017)
DOI: 10.1103/PhysRevE.96.052106
arXiv: 1708.07197

Microscopic heat engines are microscale systems that convert energy flows between heat reservoirs into work or systematic motion. We have experimentally realized a minimal microscopic heat engine. It consists of a colloidal Brownian particle optically trapped in an elliptical potential well and simultaneously coupled to two heat baths at different temperatures acting along perpendicular directions. For a generic arrangement of the principal directions of the baths and the potential, the symmetry of the system is broken, such that the heat flow drives a systematic gyrating motion of the particle around the potential minimum. Using the experimentally measured trajectories, we quantify the gyrating motion of the particle, the resulting torque that it exerts on the potential, and the associated heat flow between the heat baths. We find excellent agreement between the experimental results and the theoretical predictions.

Non-Boltzmann Distributions and Non-Equilibrium Relations in Active Baths published in Phys. Rev. E

Non-Boltzmann stationary distributions and non-equilibrium relations in active baths
Aykut Argun, Ali-Reza Moradi, Erçağ Pinçe, Gokhan Baris Bagci, Alberto Imparato & Giovanni Volpe
Physical Review E 94(6), 062150 (2016)
DOI: 10.1103/PhysRevE.94.062150

Most natural and engineered processes, such as biomolecular reactions, protein folding, and population dynamics, occur far from equilibrium and therefore cannot be treated within the framework of classical equilibrium thermodynamics. Here we experimentally study how some fundamental thermodynamic quantities and relations are affected by the presence of the nonequilibrium fluctuations associated with an active bath. We show in particular that, as the confinement of the particle increases, the stationary probability distribution of a Brownian particle confined within a harmonic potential becomes non-Boltzmann, featuring a transition from a Gaussian distribution to a heavy-tailed distribution. Because of this, nonequilibrium relations (e.g., the Jarzynski equality and Crooks fluctuation theorem) cannot be applied. We show that these relations can be restored by using the effective potential associated with the stationary probability distribution. We corroborate our experimental findings with theoretical arguments.

Aykut Argun starts his PhD

Aykut Argun starts his PhD at the Physics Department of the University of Gothenburg on 1 December 2017.

He has a Master degree from the Physics Department of Bilkent University with a Master thesis on the experimental study of thermodynamics in active baths.

He will now work on his PhD thesis on the experimental study of nanothermodynamics.

Better Stability with Measurement Errors published in J. Stat. Phys.

Better stability with measurement errors
Aykut Argun & Giovanni Volpe
Journal of Statistical Physics 163(6), 1477—1485 (2016)
DOI: 10.1007/s10955-016-1518-8
arXiv: 1608.08461

Often it is desirable to stabilize a system around an optimal state. This can be effectively accomplished using feedback control, where the system deviation from the desired state is measured in order to determine the magnitude of the restoring force to be applied. Contrary to conventional wisdom, i.e. that a more precise measurement is expected to improve the system stability, here we demonstrate that a certain degree of measurement error can improve the system stability. We exemplify the implications of this finding with numerical examples drawn from various fields, such as the operation of a temperature controller, the confinement of a microscopic particle, the localization of a target by a microswimmer, and the control of a population.